$$\int_0^\infty \frac{\log x}{ x^3-1}dx = \frac{4\pi^2}{27}$$
$$\text{Pr}\int_0^\infty \frac{dx}{x^3-1} = -\frac{\sqrt{3}\pi^2}{9}$$
$$f(z) = \frac{(\log z)^2 }{z^3-1}$$
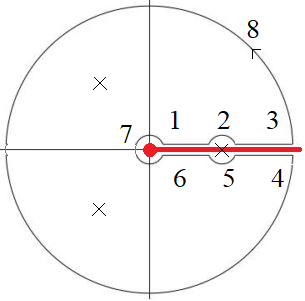
$z= 1, e^{i 2\pi/3}, ~e^{i 4\pi/3}$은 $f(z)$의 simple pole이고, $z=0$은 branch point이다. cutline은 $+x$으로 잡자. 그러면 $0\le \arg(z) \le2\pi$로 선택할 수 있다. 그리고 $z=1$은 cut line에 위치하므로 그림처럼 우회하자($C_2$와 $C_5$)
$$ \int_{C_1 + C_3} = \int_0^ \infty \frac{(\log x)^2 }{x^3-1} dx $$
\begin{align}\int_{C_4+C_6}& = \int_\infty^0 \frac{(\log x + {i2\pi})^2}{x^3-1}dx\\ &= - \int_0^\infty \frac{(\log x)^2}{x^3-1}dx +4\pi^2 \int_0^\infty \frac{1} {x^3-1}dx -i 4 \pi \int_0^\infty \frac{\log x}{x^3-1}dx\end{align}
$C_2$에서는 $z= 1+ \epsilon e^{ i \theta}~(\theta:\pi\to 0)$이므로
\begin{gather} z= \sqrt{ 1+ \epsilon^2 + 2\epsilon \cos \theta} e^{i\varphi}, \tan\varphi = \frac{\epsilon \sin \theta}{1+\epsilon \cos \theta} \\ \log(z) = \log\sqrt{1+ \epsilon^2+2\epsilon \cos \theta } + i \varphi\to 0\\ dz = i \epsilon e^{i \theta } d \theta \\ z^3-1 = 3\epsilon e^{i \theta}(1+ O(\epsilon)) \\ \int_{C_2} \longrightarrow 0\end{gather}
$C_5$에서는 $z= e^{i2\pi } + \epsilon e^{i \theta} ~(\theta: 2\pi \to \pi)$이므로
$$\int_{C_5} = \int_{2\pi}^\pi \frac{ [\log(e^{i2\pi}+ \epsilon e^{i\theta}) ]^2 }{3\epsilon e^{i \theta}(1+ O(\epsilon)) } i \epsilon e^{i \theta} d \theta = -i \frac{4\pi^2}{3}$$
그리고 $C_7$과 $C_8$에서 적분은 0으로 수렴함을 쉽게 알 수 있다. $z= e^{i 2\pi/3}, e^{i4\pi/3}$에서 residue는 각각 $$ \text{Res}(e^{i 2\pi/3}) = \frac{[\log (e^{i 2\pi/3})]^2 }{ (e^{i2\pi/3}- 1) (e^{i2\pi/3} - e^{i 4\pi/3}) } = \frac{2\pi^2}{27} (1- i \sqrt{3})$$ $$ \text{Res}(e^{i 4\pi/3}) = \frac{[\log (e^{i 4\pi/3})]^2 }{ (e^{i4\pi/3}- 1) (e^{i4\pi/3} - e^{i2\pi/3})} = \frac{8\pi^2}{27} (1+ i \sqrt{3})$$
또 $x=1$에서 $$\frac{\log x}{x^3-1} = \frac{1}{3} -\frac{1}{2}(x-1) + \frac{1}{2} (x-1)^2 + ... $$이므로 $x=1$이 removable singularity 이지만, $\frac{1}{x^3-1}$에 대해서는 isolated singularity이므로 principle value을 취해야 한다. 이제 residue 정리에 의해서
$$ 4\pi^2 \text{Pr} \int_0^\infty \frac{dx}{x^3-1} -i 4\pi \int_0^\infty \frac{\log(x) dx}{x^3-1} + i \frac{4\pi^2}{3}= i \frac{20}{27} \pi^3 -\frac{4\sqrt{3}}{9} \pi^3$$이므로 $$ \text{Pr}\int_0^\infty \frac{dx}{x^3-1} = -\frac{\sqrt{3}}{9}\pi^2 \\ \int_0^\infty \frac{\log x dx }{x^3-1}= \frac{4}{27} \pi^2$$
'Mathematics' 카테고리의 다른 글
| Integration along a branch cut-037 (0) | 2024.11.21 |
|---|---|
| Integration along a branch cut-036 (0) | 2024.11.20 |
| Integrate [log(1+x^2)/1+x^2, {x, 0, ∞}] (5) | 2024.11.15 |
| Integrate [log(1+x)/(1+x^2), {x, 0, 1}] (0) | 2024.11.15 |
| Integrate [log(1+x)/x, {x, -1, 1}] (0) | 2024.11.13 |




