Geometry & Recognition

표준형 타원

$$ \frac{x^2}{a^2} + \frac{y^2}{b^2} = 1$$

의 두 주축에 대한 central moment (moment of inertia)는 각각

$$ \mu_{20} = \frac{\pi}{4} a^3 b, \quad \mu_{02} = \frac{\pi}{4} ab^3$$ 

으로 주어짐은 쉽게 계산할 수 있다. 그런데 타원의 면적인 0차 central moment가 $\mu_{00}= \pi ab$이므로 normalized central moment (위치의 분산을 의미한다)는 각각

$$\tilde\mu_{20} = \frac{\mu_{20}}{\mu_{00}} = \frac{1}{4}a^2, \quad  \tilde\mu_{02} = \frac{\mu_{02}}{\mu_{00}} = \frac{1}{4}b^2$$

따라서 장축과 단축의 반지름은 주축에 대한 2차 normalized central moment을 구하면 얻을 수 있다.

기울어진 타원의 경우는 $\tilde\mu_{pq}$가 픽셀의 분포를 알려주므로 이를 이용한 covariance 행렬의 고유값을 구하면 장축과 단축의 반지름을 알 수 있고, 기울어진 정도도 알 수 있다. 공변행렬

$$ \Sigma = \left( \begin{array}{cc} \tilde\mu_{20} & \tilde\mu_{11} \\ \tilde\mu_{11} & \tilde\mu_{02} \end{array} \right)$$

의 두 고윳값은

$$ \lambda_1 = \frac{1}{2}\left( \tilde\mu_{20}+ \tilde\mu_{02} + \sqrt{ (\tilde\mu_{20} - \tilde\mu_{02})^2 + 4 \tilde\mu_{11}^2} \right),$$

$$ \lambda_2 = \frac{1}{2}\left( \tilde\mu_{20}+ \tilde\mu_{02} - \sqrt{ (\tilde\mu_{20} - \tilde\mu_{02})^2 + 4 \tilde\mu_{11}^2} \right).$$

따라서 기울어진 타원의 장축과 단축의 반지름, 그리고 회전각은

$$ a = 2\sqrt{ \lambda_1}, \quad b = 2\sqrt{ \lambda_2}, \quad \tan(2\theta)=\frac{2 \tilde\mu_{11}}{ \tilde\mu_{20}-\tilde\mu_{02}   }$$

로 주어지므로 회전된 좌표계 $(u, v)$에서 타원의 방정식은

$$ \frac{ u^2 }{4 \lambda_1} + \frac{v^2 }{4\lambda_2} = 1$$

로 쓰인다. 

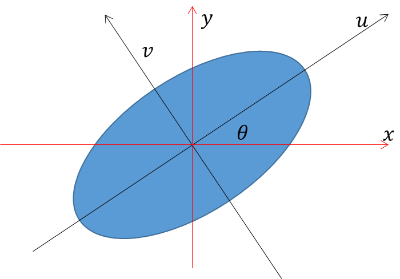
다시 원 좌표계 $(x, y)$로 돌아가기 위해서 (물론 질량중심이 원점인 좌표계이다)

$$ u =  \cos \theta x+  \sin \theta y, \quad v = -\sin \theta x + \cos \theta y$$

를 대입하면 타원을 2차식 형태

$$ A x^2 + 2B xy + C y^2 = 1$$

로 쓸 수 있는데, 그 계수는

$$ A= \frac{1}{8}\left( \frac{1}{\lambda_1} + \frac{1}{\lambda_2} +\Big( \frac{1}{\lambda_1} - \frac{1}{\lambda_2} \Big) \cos (2\theta) \right)=\frac{\tilde\mu_{02}}{ 4(\tilde\mu_{20}\tilde\mu_{02}-\tilde\mu_{11}^2 )} $$

$$ C= \frac{1}{8}\left( \frac{1}{\lambda_1} + \frac{1}{\lambda_2} -\Big( \frac{1}{\lambda_1} - \frac{1}{\lambda_2} \Big) \cos (2\theta) \right)=\frac{\tilde\mu_{20}}{ 4(\tilde\mu_{20}\tilde\mu_{02}-\tilde\mu_{11}^2 )} $$

$$ B= \frac{1}{8}\left( \frac{1}{\lambda_1} - \frac{1}{\lambda_2} \right)\sin (2\theta)=\frac{-\tilde\mu_{11}}{ 4(\tilde\mu_{20}\tilde\mu_{02}-\tilde\mu_{11}^2 )} $$

로 주어진다. 주어진 object의 normalized central moment를 구하면 타원 fitting에 대한 정보를 구할 수 있음을 보였다.

영상처리에서 영상에서 분리된 객체의 형상을 기술할 때 moment를 많이 사용한다. 영상은 각 픽셀 위치에서 컬러 값이 할당된 일종의 2 변수 함수로 생각할 수 있다. 영상의 모멘트는 픽셀 위치(의 단항식)를 컬러값으로 가중치를 주어서 낸 평균이라고 간단하게 기술할 수 있다.  이미지가 $I(x, y)$로 주어진 경우 $p+q$-차 moment는

$$m_{pq} = \iint x^p y^q I(x, y) dxdy, \quad p, q=0,1,2,3...$$

로 정의한다. 그러나 이 정의는 똑같은 형상이라도 원점에서 얼마나 떨어진 위치에 있는가에 따라 결과가 달라지므로 (원점의 선택에 의존되는 정의다. 객체를 구성하는 픽셀 개수를 알려주는 $m_{00}$는 불변이다.) 형상 기술에 적합한 형태가 아니다. 이 문제는 객체의 질량중심에 대한 상대 위치에 대한 weighed mean인 central moment를 feature 값으로 이용하면 된다:

$$ \mu_{pq} = \iint (x - \overline{x})^p ( y - \overline{y})^q I(x, y) dx dy$$

여기서 

$$\overline{x} = m_{10}/m_{00}, \quad \overline{y} = m_{01}/m_{00}$$

로 객체의 질량중심(center of mass)의 위치다. 0차 central moment는 원래의  moment와 같고, 1차 central moment는 질량중심이 원점이므로 0으로 주어진다: $\mu_{10}=\mu_{01}=0$. 이 central moment는 이미지에서 객체가 이동하더라도 동일한 값을 가진다.

그런데 같은 객체를 담은 이미지라도 확대하거나 축소하면 central moment의 값은 변하게 되므로 영상의 scale이 달라지는 경우는 central moment를 feature로 한 형상 판별하는 작업에는 적당하지 않을 수 있다. 이미지를 전체적으로 축소하거나 확대하더라도 같은 값을 주는 scale invariant인 moment 정의가 필요한데, 이는 central moment를 정규화시켜 사용하면 된다.

우선 이미지를 $\lambda$ 만큼 확대하거나 축소하면 변환된 이미지는 $I'(x', y') = I(x'/\lambda, y'/\lambda)$로 표현된다. 이 이미지에서 central moment를 계산하면

\begin{align} \mu'_{pq} &= \iint (x'- \overline{x'})^p ( y' - \overline{y'})^q I(x'/\lambda , y'/\lambda ) dx'dy'\\ &= \lambda^{p+q+2} \iint (x- \overline{x} )^p(y -\overline{y})^q I(x, y) dx dy \\ &= \lambda^{p+q+2} \mu_{pq} \end{align}

즉, 이미지를 scaling 하면 $\mu_{pq}$는 scaling factor $\lambda$의 ${p+q+2}$ 지수승만큼 변하게 된다. 이 변화를 없애기 위해서 양변을 제일 단순한 central moment인 $\mu_{00}^\gamma$로 나누어서 전체적인 scale factor가 사라지게 만들자. 그런데 $$\mu'_{00} = \lambda ^2 \mu_{00}$$

이므로

$$ \frac{ \mu'_{pq} }{ (\mu'_{00})^\gamma} = \lambda^{p+q+2-2\gamma} \frac{\mu_{pq}}{ (\mu_{00})^\gamma } $$

$\gamma$를

$$ \gamma = \frac{p+q}{2}+1$$

로 선택하면 다음의 normalized central moment는 scaling에 대해서 불변인 성질을 가진다.

$$ \eta_{pq} = \frac{\mu_{pq}}{\mu_{00}^\gamma}$$

이진 이미지에서 지름 $2a$인 원과 한 변이 $2a$인 정사각형에 대해서 2차 central moment을 구해보면

$$\text{circle: }~  \mu_{20}= \mu_{02}=\frac{\pi}{4} a^4 = 0.7854 a^4 ,~~\mu_{11}=0,$$

$$\text{rectangle: }~\mu_{20}=\mu_{02}=\frac{4}{3} a^4 = 1.3333 a^4, ~~\mu_{11}=0.$$

그리고 $\mu_{00}= \pi a^2~(\text{circle})$, $\mu_{00}= 4 a^2 ~(\text{rectangle})$이므로 2차 normalized central moment는

$$\text{circle: }~ \eta_{20}=\eta_{02} = \frac{1}{4\pi} = 0.07956,\quad \eta_{11}=0, $$

$$\text{rectangle:}~\eta_{20}=\eta_{02}=\frac{1}{12}=0.08333,\quad \eta_{11} = 0.$$

normalized central moment는 크기에 의존하지는 않지만 원과 사각형에서 분명한 차이를 보이므로 이미지에서 분리된 객체를 구별하는 기준으로 삼을 수 있을 것이다.

물론 normalzed central moment는 객체를 회전시키면 그 값이 다시 변하게 된다. 하지만 이들을 잘 조합하면 회전 불변인 성질까지 추가되는 invariant moment를 구성할 수 있다. 이차 central moment의 경우 질량중심에 대한 회전변환을 고려할 때 서로 다르게 변환하는 부분이 섞여 있는데 이를 분리하면

\begin{gather} \mu_{20}= \iint \left[ \frac{1}{2}(x^2-y^2) + \frac{1}{2}(x^2+y^2) \right] f(x, y) dxdy =T_{11} +\delta_{11} S ,\\ \mu_{11}= \iint xy I(x, y) dxdy = T_{12}+\delta_{12}S, \\ \mu_{02} = \iint \left[ -\frac{1}{2}(x^2 - y^2) +\frac{1}{2}(x^2+y^2) \right] dx dy= T_{22} + \delta_{22} S ,\\ T_{ij} = \iint \left[x_i x_j -\frac{1}{2}(x^2+y^2) \delta_{ij} \right] I(x, y) dxdy=\frac{1}{2} \left(\begin{array}{cc}\mu_{20} - \mu_{02} & 2\mu_{11} \\ 2\mu_{11}& -(\mu_{20}-\mu_{02}) \\\end{array} \right),\\ S= \iint (x^2 + y^2) I(x, y)dx dy =\mu_{20}+\mu_{02}   \end{gather}

로 쓸 수 있는데, $T_{ij}$는 회전 변환에 대해서 rank가 2인 tensor의 성분을 구성하고, $S$는 $rank=0$인 scalar가 된다.  traceless인 $rank=2$인 텐서를 가지고 만들 수 있는 회전에 대해 invariant 한 양은 tensor의 determinant ($\det(T)$)인데, 이를 normalized central moment로 표현하면 (scaling까지 고려하기 위해서)

$$ \text{det}(T) \propto (\eta_{20} - \eta_{02})^2 + 4 \eta_{11}^2 \quad (\text{up to a numerical factor})$$

로 쓸 수 있고, $rank = 0$ 인 부분($S$)은 그 자체로 회전에 불변이다. (물리적으로는 질량중심축에 대한 rotational inertial에 대응하는 양이다). 

$$ \eta_{20} + \eta_{02}= \frac{1}{\mu_{00}^2} \iint (x^2 + y^2 ) I(x, y) dxdy$$

다른 회전 invariant 한 양은 order가 3 이상인 central moment를 이용해서 만든 rank=3 이상인 tensor의 invariant을 이용해서 얻을 수 있다.