$$ I = \int_0^1 \frac{\log(1+x)}{1+x^2} dz =\frac{\pi}{8} \log{2}$$
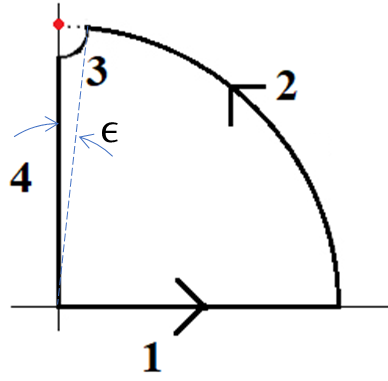
함수 $$f(z)= \frac{\log(1+z)}{1+z^2}$$을 그림과 같은 경로을 따라 적분을 하자.
경로 1에서
$$ \int_\text{path-1} = I$$
경로 2에서 $z= e^{i \theta} ~(\theta:0 \to \pi/2)$
$$ 1+ e^{2i\theta} = 2\cos \theta e^{i \theta} \\ \log(1+e^{i \theta} ) = \frac{1}{2} \log (2+ 2\cos \theta) e^{i \theta} = \frac{1}{2} \log(2 + 2\cos \theta) + i \theta$$
$$\int_\text{path-2} = \frac{i}{4} \int_0^{\pi/2-\epsilon} \frac{\log(2+ 2\cos \theta)d\theta}{\cos \theta} -\int_0^ {\pi/2-\epsilon}\frac{\theta d\theta}{\cos \theta}$$
경로 3에서 $z= i + \epsilon e^{i \theta}$이므로
$$ \int_\text{path-3} = \int_0^{-\pi/2} \frac{\log( 1+ i + \epsilon e^{ i \theta}) }{\epsilon^2 e^{2i \theta} + 2i \epsilon e^{i \theta}} i \epsilon e^{i \theta} d \theta \to -\frac{\pi}{8} \log(2) - i \frac{\pi^2}{16}$$
경로 4에서는 호의 반지름이 $\epsilon$임을 고려하면 $ z = i y (y: 1-\epsilon\to 0)$이므로
$$\int_\text{path-4} = \int_{1-\epsilon}^0 \frac{\log(1+ iy) }{1-y^2} idy = \int_0^{1-\epsilon} \frac{-\frac{i}{2}\log(1+y^2) + \tan^{-1} (y) }{1-y^2}dy$$
폐경로 내부에서 $f(z)$가 analytic하므로 $\oint f(z) dz=0$이고, 실수부는
$$ I = \frac{\pi}{8} \log(2) + \int_0^{\pi/2-\epsilon} \frac{\theta d\theta}{4\cos \theta} - \int_0^{1-\epsilon} \frac{\tan^{-1}(y) dy}{1-y^2}$$ 두번째와 세번째의 적분은 동일하게 logarithmic하게 발산하므로 그 차이는 유한한 값을 가질 수 있다.
$$ \theta =\frac{\pi}{2}-\epsilon: ~ \frac{\theta}{4\cos \theta} = \frac{\pi/2}{4 \epsilon}=\frac{\pi}{8\epsilon}$$
$$ y=1-\epsilon:~ \frac{\tan^{-1}(y)}{1-y^2}= \frac{\pi/4}{2\epsilon} = \frac{\pi}{8\epsilon}$$ 그리고 $\tan \theta/2=y$로 치환을 하면 $\cos \theta = \frac{1-y^2}{1+y^2}$이므로 두번째 항과 세번째 항이 상쇄됨을 확인할 수 있다. 허수부는
$$ \frac{1}{4}\int_0^{\pi/2} \frac{\log (2+ 2\cos \theta) d \theta}{\cos \theta} - \frac{\pi^2}{16} -\frac{1}{2}\int_0^1\frac{\log (1+y^2)dy}{1-y^2} = 0$$ 마찬가지로 위에서와 같이 치환을 하면 등호가 성립함을 보일 수 있다.
'Mathematics' 카테고리의 다른 글
| Integration along a branch cut-035 (0) | 2024.11.19 |
|---|---|
| Integrate [log(1+x^2)/1+x^2, {x, 0, ∞}] (5) | 2024.11.15 |
| Integrate [log(1+x)/x, {x, -1, 1}] (0) | 2024.11.13 |
| Integration along a branch cut-034 (0) | 2024.11.10 |
| 도넛의 표면적, 부피 (1) | 2024.11.10 |




