Integration along a branch cut-011

$$I= \int_c^\infty \frac{dx}{x^2 - 1} \quad(c > 1)$$

복소함수 $f(z)= \frac{\log(z-c) }{ z^2 -1}$을 그림의 contour을 따라 적분을 한다. $z=c, \infty$가 branch point이므로 $x>c$인 $x$축을 따라 cut line을 설정한다. 또, $z=\pm 1$은 simple pole이다.

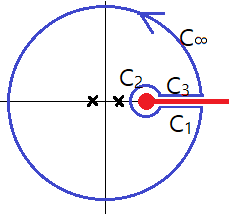
$C_2$:  $x-c=\epsilon e^{i\theta}$로 매개화하면 $$ \int f(z) dz = O( \log(\epsilon) \epsilon ) \rightarrow 0.$$$C_1$: $z-c = (x-c) e^{i 2\pi}~(x: \infty \rightarrow c)$이므로,$$ \int_{C_1} f(z) dz = \int_\infty ^c \frac{1}{x^2 -1} ( \log (x-c) + 2i \pi) dx $$$C_3$: $z-c= (x-c) e^{i 0} ~(x: c \rightarrow \infty)$이므로,$$ \int_{C_3} f(z) dz = \int_c^\infty \frac{1}{x^2 - 1} \log(x-c) dx $$ $C_\infty$: $z = Re^{i \theta}$로 매개화하면, $$\int f(z) dz  = O( \log(R) /R ) \rightarrow   0$$ 따라서, $$\int_{\Gamma}  f(z) dz= 2 i \pi  [\text{Res}(z=1) + \text{Res}(z=-1)] =2i \pi \left( \frac{\log (c-1)}{2} - \frac{ \log(c+1)}{2}\right)$$

정리하면,

$$\int_c^\infty \frac{dx}{x^2 -1} = \log\sqrt{\frac{c+1}{c-1}}$$

물론 $\frac{1}{x^2-1} = \frac{1}{2} (\frac{1}{x-1} - \frac{1}{x+1})$임을 이용하는 것이 더 쉽다.