$$I = \int_{-\infty}^\infty \frac{p e^{ipr} dp}{\sqrt{p^2 + m^2 } } \quad (r>0,~m>0)$$
복소함수 $$f(z) =\frac{z e^{izr} }{\sqrt{z^2 + m^2}}$$의 contour 적분을 이용한다. $z=\pm im$가 $f(z)$의 branch point이므로 그림과 같이 cut line을 잡는다(적분은 upper half plane에서 한다). 위상은 $$-\frac{3\pi}{2} \le \arg(z-im)\le \frac{\pi}{2},\quad -\frac{\pi}{2}\le \arg(z+im) \le \frac{3\pi}{2}$$로 선택한다.
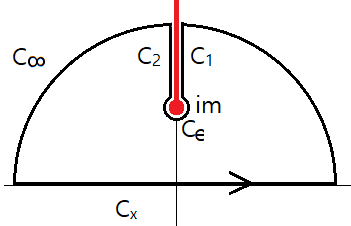
그림의 contour에서 $f(z)$가 analytic하므로 $$\int_\Gamma f(z) dz = 0$$이고, $C_1$: $z-im = (s-m) e^{i \pi/2} ~~(s: \infty\rightarrow m)$, $z+im = (s+m) e^{i\pi/2}$, $z=is$이므로 $$\int_{C_1} f(z) dz = \int_{\infty}^m \frac{ (is) e^{-sr} (ids)}{ \sqrt{s^2 - m^2 } e^{i\pi/2}} = -i \int_m^\infty \frac{s e^{-sr} ds }{ \sqrt{ s^2 - m^2}} .$$
$C_2$: $z-im= (s-m) e^{-i 3\pi/2} ~~(s: m\rightarrow \infty)$, $z+im = (s+m) e^{i \pi/2}$, $z=is$이므로
$$\int_{C_2} f(z) dz = \int_m^\infty \frac{ (is) e^{-sr} (ids) }{ \sqrt{s^2 - m^2 }} e^{-i \pi /2} = -i \int_m^\infty \frac{s e^{-sr}ds }{\sqrt{s^2 - m^2} }.$$
그리고 $C_\epsilon:~ z=\epsilon e^{i \theta}$에서는 $$ \int_{C_\epsilon} f(z) dz = O( \sqrt{\epsilon})\rightarrow 0.$$
$C_\infty$에서 $z=R e^{i\theta} ~(\theta:0\rightarrow \pi)$로 놓으면 $|e^{izr}| \le e^{- R \sin \theta r} \rightarrow 0$이므로
$$\int_{C_\infty} f(z) dz \rightarrow 0.$$
따라서 $x$-축을 따라 적분한 값 $I$는
$$I = \int_{-\infty}^{\infty} \frac{p e^{i p r}dp}{ \sqrt{p^2 + m^2 }} = 2i \int_m^\infty \frac{se^{-sr} ds} {\sqrt{s^2 - m^2}} =i\times \text{positive number}.$$ 우변의 적분은 Modified Bessel function of the second kind $$I=2i \times m K_1(mr)$$로 표현이 된다.
'Mathematics' 카테고리의 다른 글
| Integration along a branch cut-011 (0) | 2021.01.04 |
|---|---|
| Integration along a branch cut-010 (0) | 2021.01.04 |
| Integration along branch cuts-008 (0) | 2021.01.03 |
| Integration along a branch cut-007 (1) | 2020.12.31 |
| Integration along a branch cut-006 (0) | 2020.02.28 |




