$$I= \int_0^\infty \frac{ \log x dx }{x^3-1}= \frac{4}{27} \pi^2$$
함수 $$f(z) = \frac{\log z}{z^3 - 1}$$을 그림과 같은 경로에 적분하자.(참고: https://kipl.tistory.com/670)
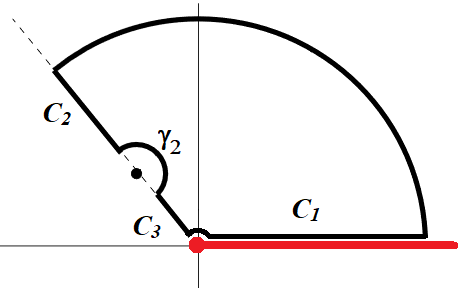
$z=0$은 branch point이므로 그림과 같이 cutline을 선택한다. 그리고 $z=1$은 removable singularity이므로 별다른 처리가 필요없고( $\frac{\log x}{x^3-1} = \frac{1}{3} -\frac{1}{2}(x-1) + \frac{1}{2} (x-1)^2 + ... $). $C_\infty$와 $z=0$을 감싸는 $\gamma_1$에서 적분은 0에 수렴한다. $z=e^{i 2\pi/3} $은 simple pole로 경로 $\gamma_2$처럼 우회한다. 그러면 경로 $\gamma_2$에서 $z= e^{i2\pi/3} + \epsilon e^{i \theta}$이므로 $$ \int_{\gamma_2} = \int_{2\pi/3}^{-\pi/3} \frac{ \log ( e^{i 2\pi/3} ) (i \epsilon e^{i \theta} d \theta ) }{ \epsilon e^{i \theta} ( e^{i2\pi/3} - 1)(e^{i2\pi/3}- e^{i 4\pi/3})} = \frac{2\pi^2}{9} e^{i 2\pi/3}$$ $C_1$에서는 $z= x~(x: 0 \to \infty)$이므로 $$ \int_{C_1} f(z) dz = I$$ $C_2 +C_3$에서는 $z= e^{i 2\pi/3} x = e^{i 2\pi/3}x~(x:\infty \to 0)$이므로 \begin{gather} \int_{C_2+C_3} = \int_\infty ^0 \frac{ \log (x e^{i 2\pi/3} ) e^{i 2\pi/3} dx}{ x^3 -1}= - e^{i2\pi/3}\int_0^\infty \frac{\log x +i\frac{2\pi}{3}}{x^3-1}dx\\ =- e^{i 2\pi/3} \left( I + i \frac{2\pi}{3} \int_0^ \infty \frac{dx}{x^3-1} \right) = - e^{i 2\pi/3} \left( I + i \frac{2\pi}{3} J \right) \end{gather} $J = \int_0^\infty \frac{dx}{x^3-1} =- \frac{\sqrt{3}}{9} \pi$ 이므로(https://kipl.tistory.com/670) \begin{gather} \oint f(z) dz = 0 ~\to ~(1- e^{i 2\pi/3}) I -i \frac{2\pi}{3} e^{i 2\pi/3} J + \frac{2\pi^2 }{9} e^{i 2\pi/3}=0 \\ I = \frac{e^{i 2\pi/3}}{1- e^{i 2\pi/3}} \left( -i \frac{2\pi}{3} J - \frac{2\pi^2}{9} \right) = \frac{4}{27}\pi^2 \end{gather}
'Mathematics' 카테고리의 다른 글
| Integration along a branch cut-040 (0) | 2024.11.24 |
|---|---|
| Integration along a branch cut-039 (0) | 2024.11.23 |
| Integration along a branch cut-037 (0) | 2024.11.21 |
| Integration along a branch cut-036 (0) | 2024.11.20 |
| Integration along a branch cut-035 (0) | 2024.11.19 |




